Low Resolution Spectrography

Spectrography is one of the key disciplines of astronomy, perhaps even the most significant. Indeed, the essence of our physical knowledge of the stars comes from the spectral analysis of light which we collect with our telescopes. However spectrography is not well thought of by amateur astronomers. It has the reputation of a difficult, dry, inaccessible technique. The few images which follow show that it is nothing of the sort if the subject is well approached and if one retains modest performance expectations. This study was carried out within the framework of the Audine program with an aim of producing a very luminous spectrograph to carry out multispectral photometry of supernovas, quasars and comets, with amateur telescopes.

The majority of spectrographs use a beam splitter. This optical component has the property of bending light which crosses it to different angles depending on wavelength. The best-known beam splitter is the prism. The mechanism of dispersion is the variation of the angle of refraction which is a function of the index of refraction of glass, which itself varies depending on wavelength. It is also refraction which explains rainbows, the light in this case being bent in a complex way by the water drops of a downpour.

The other significant family of beam splitters is based on light diffraction when it crosses a fine periodic structure or is reflected on a structure of this type. These are diffraction gratings. The coloured irisations observed in the light reflected by a compact disc originate in a diffractive phenomenon, the periodic structure being here the fine furrow left by the laser which engraved the disc.

The simplest spectrograph that one can build uses the only beam splitter which one places directly in the convergent optical beam of the telescope, near the focal plane, i.e. CCD sensor. No need for slit, collimator and other objective to generate a completely suitable spectrum. This simplification has of course a price. A spectrograph based on a beam splitter in the convergent beam cannot claim to compete as regards resolution with the more traditional assembly of the spectrograph with slit. On the other hand the ease of realization is beyond measure.

The figure below shows how to lay out the beam splitter, here a grating, in the optical beam. The polychromatic light coming from a star in the field is bent while crossing the grating to form a spectrum in the plane of the CCD. In the figure only 3 rays of light corresponding to 3 distinct wavelengths are traced. In practice, the number of wavelengths is infinite and a continuous spectrum is observed. Another star in the field will produce a spectrum in the same manner, but this one will be shifted spatially. It will be the same for all the other objects in the field: galaxies, asteroids, novae... It is seen that this spectrograph makes it possible simultaneously to acquire the spectra of a very great number of objects, and that is not the least of its advantages. Comparatively, a spectrograph with slit will allow only one object to be studied at the same time.

Placement of the beam splitter in the converging beam of the telescope
 
The following figure shows that on both sides of the zero-order image of the star Vega (saturated on this document, which caused the blooming), are formed the spectra of orders 1 and -1.

Farther still one finds higher orders, but they are too weak to give a visible signal in this reproduction of the CCD image. The orders cause artifacts because of their recovery. For example, the wavelength 8000 A in order 1 is superimposed on the wavelength 4000 A in order 2. As a simplification, it should be considered that the spectrum with order 1 is not exploitable beyond 8000 A because of this phenomenon with the CCD. The grating is a simple photographic Cokin N°40 special effect filter placed 40 mm in front of the Audine camera.

The system of spectra produced by a diffraction grating
Negative image of a star field observed by interposing a grating with transmission in the optical beam. Filter Cokin N°40 and telescope of 190 mm to F/d=4. Notice in this image two brilliant stars. On right-hand side of each one you have a horizontal line. You see there the spectrum of order 1 of these two stars. The roughly specific images correspond with order zero; the images which you would observe same as if you had not set up the grating. All stars of this field produce spectra but the others are too weak to be visible in this reproduction. Note that the spectra are relatively thin, a sign which they are clean, but at the same time, the stars are rather strongly unfocused at order 0, which betrays a curve of field, inherent in this type of assembly. By examining the spectra carefully you can already see some spectral lines.
 
Once the image of the spectrum obtained, you must graph the spectral profile (by using for example the software VisualSpec or QMiPS32).
 
The spectrum of the star Vega. The blue part of the spectrum is on the left, the red part is on the right. The principal spectral lines are marked. One notices the particularly clear hydrogen series in stars of type A0. The infra-red part is made conspicuous by the presence of the molecular bands of gas components of the Earth's atmosphere: band B of O2 between 6850 and 7020 A, band H20 between 7000 and 7400 A, and band A of O2 between 7580 and 7750 A.

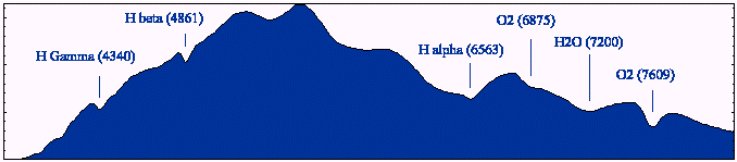
The spectrum of the figure above is limited spectrally by the response of the CCD. On left the spectrum begins in blue from approximately 3900 A. On right-hand side, the spectrum disappears in the infra-red around 1 micron wavelength (in the figure the spectrum is voluntarily stopped in the neighbourhood of 8000 A because of the problem of recovery of order). Between these two end points the curve varies in a complex manner, and many variations of intensity in the spectrum must originate with the CCD itself. A significant part of processing of spectra consists in eliminating this contribution of the detector, but also that of the transmission of optics or that of the output of the grating which also varies with wavelength.

Excellent diffraction gratings are distributed by the Jeulin company (specialized in the supply of scientific equipment for National Education). They are prepared in slide holders out of glass. A fundamental property of these gratings is that they concentrate a large part of the optical flux in only one order (one calls that the blaze). The output of the spectrograph is thus very appreciably increased. The slide holders slip without problems into the carry-filters of Association Aude. One sees in this image one of the last prototypes of the Audine camera (the case is not yet black anodized).

The image below shows the area of planetary nebula NGC 2392 (the Clown nebula) obtained with a Jeulin grating with 100 lines/mm placed 21 mm in front of the significant surface of KAF-0400 CCD of the Audine camera. The telescope is a flat-field camera of 190 mm to F/d=4. The final image is a composite of 10 2-minute exposures.

The area of planetary nebua NGC 2392. Order zero of this one is just at the right of center in the image (one can see the nebular halo). Notice that a spectrum is associated with each star. That of nebula is complex: one sees at the same time the spectrum of the central star, which is approximately magnitude 9, and several monochromatic images of nebula which are superimposed on it. The Jeulin grating of 100 lines/mm concentrates approximately 40% of the signal in order 0, 40% in order 1 and 20% in the -1 and higher orders.
Examples of spectral profiles obtained with the Jeulin grating of 100 lines/mm placed at the opening of a telescope 190 mms in diameter. The spectrum in green is that of the star Delta Cassiopée, of type A5. The Balmer series of hydrogen is quite visible. In red, one finds the spectrum of the star Gamma Cassiopia, of type B0e. The line of hydrogen H alpha appears there in emission very strongly. The exposure times are 30 seconds. One sees here how a grating costing about 100 Fr ($13 USD), placed simply at a few centimetres in front of the camera, makes it possible to perform good physics!
The spectrum of a very red star (SAO5932). This one displays multiple molecular bands. The weaker star, located just below, presents a more traditional spectrum with a quite uniform continuum. Telescope of 190 mm with the grating 100 lines/mm is placed very close to the CCD, at 19.46 mm, so as to have maximum luminosity. This is possible thanks to the compactness of the Audine camera.
Spectral profile of star SAO5932. The spectrum begins with 0.443 micron on the left and ends with 0.990 micron on the right.
Our quick and handy small spectrograph gives access the spectrum of galaxies. One can see on this image, carried out with the 190 mm telescope (composite of 19 2-minutes exposures), elliptic galaxies NGC 2258 on the right, and NGC 2256 on the left (the galaxies are surrounded by a blue rectangle). The spectra of these galaxies are surrounded by an orange rectangle. They resemble those of the majority of stars. The grating of 100 lines/mm was placed at 19.46 mm from the significant surface of the KAF-0400 and dispersion was 4Ä/pixels. North is on the right.

The spectrum of double system NGC 3690 (ARP 299) carried out with the same instrumental configuration as for the preceding image. It is a composite of 37 images exposed 120 seconds, that is to say a cumulative time of integration of 1 hour and 10 minutes. The spectrum of the core of these active galaxies clearly shows an emission line (note that at the time of the exposure, 28/01/1999, a supernova of magnitude 16.5 was just on the right principal core of NGC 3690, but it was inaccessible to this spectrograph since it was too weak). The wavelength measured for the emission line is 0.6740 micron. If it is the H alpha band presenting a P-Cygni profile and normally at 0.6563 micron, but shifted towards the red because the speed of recession, then measured radial speed is of 8000 km/s whereas the galaxy is announced to have a radial speed of 3000 km/s. The variation can be explained by an erroneous identification of the band or a strong deformation of the band because of high speeds of expansion in the core. On this document it is possible to exploit star spectra up to magnitude 14.8. If the object presents emission lines, it is probably possible to go down low in magnitude. It should be stressed that in the assembly used, the spectrum appears in superposition with the background of sky. Working with a quite dark sky, it is possible to reduce the proportion of noise added by the level of the background of sky (all the observations of this page were carried out in urban environment). The estimated gain is 0.5 magnitude. By using under these conditions a telescope of 300 mm, one calculates that it is possible to acquire measurable spectra at least up to magnitude 16.5. It is seen that the objective of observation of supernovas or quasars with this simple device is not utopian.

 

Non-calibrated spectral profiles photometrically extracted from the preceding image. On the left, a normal star of the field. On right-hand side, the principal core of system ARP 299 (the emission line is quite visible). The spectral extent goes from 0.443 to 0.793 micron.
To generate this image, showing the spectrum of principal stars of the Pleiades (M45), the Jeulin grating of 100 lines/mm was placed between a simple photographic objective of 80 mm focal distance, stopped to F/5.6 and the Audine camera. The band of atmospheric atomic oxygen at 0.76 micron is quite visible in the spectrum of the majority of stars.
Spectral classification with the range of the Audine camera associated with a photographic objective with 80 mm focal distance and a raiseau (network?) with 100 lines/mm. On these images, the blue part of the spectrum is on the left. On top, the spectrum of the star Betelgeuse, which shows broad band caused by molecules being able to exist in the cold atmosphere of this star. The largest part of the energy is emitted in the infra-red. In the center, the spectrum of the star Rigel, a much hotter star. Notice how the continuum is offset towards the blue part of the spectrum. At bottom, the spectrum of the planet Jupiter, which shows strong absorption bands caused by methane, a major component of the atmosphere of the giant planet.
 
In order to know more about Audine and Spectrography, go here.